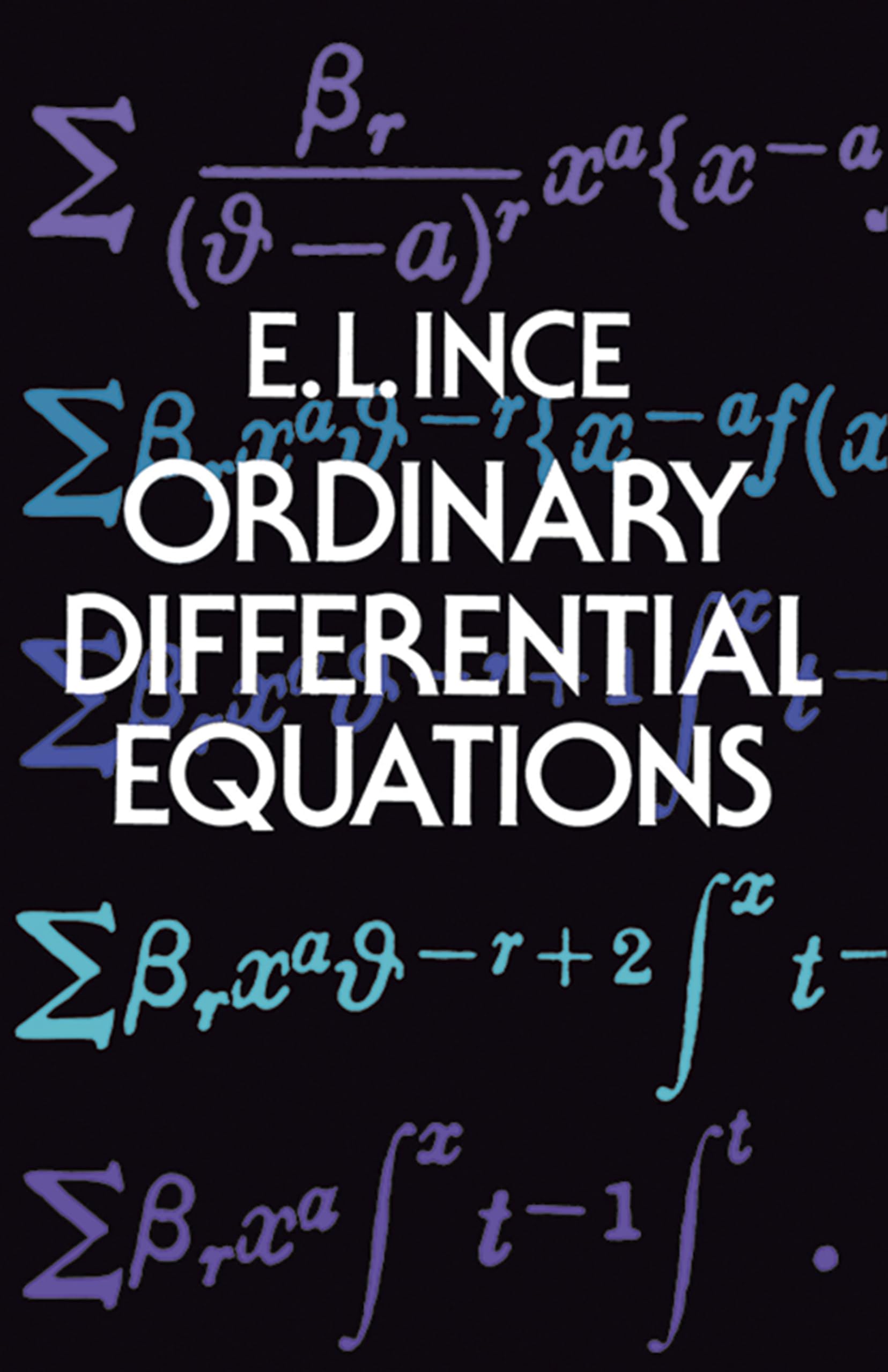
Ordinary Differential Equations (Dover Books on Mathematics)

I own more than a hundred math books, and am always on the lookout for good ones. This book has caught my eye because it presents the material in a very logical arrangements; the chapters are sorted by topics, and each chapter has its own introduction, line of argument, and presents cohesive results that build one on another. Simply an amazing book. Now on my "best math books" shelf of my bookshelf, among other renowned titles like Cassels & Frohlich, Lam, and Miranda.

This is a classic and has sections that I was particularly interested. The binding is very good. The overall condition was very good.

One could inquire as to 'Why' a text published as long ago as 1926 needs recommendation at this point in time.My college course in ordinary differential equations consisted of a potpourri of computer lab exercises coupled with odds and ends meant to tie things together. Notwithstanding the utility of a computer graphics component of the course, still an unsatisfying course for all those concerned with pedagogy of present modes of instruction (regards that course, peruse: The College Mathematics Journal, Special Issue On Differential Equations, Volume 25, Number 5, November 1994). As I reflect upon the course (1996), I ask:could the outcome have been different ? Enter the resource by E.L. Ince. You will not find graphics and illustrations adorning every page (in fact, there are almost none, as only page 62 has a diagram illustrating Lipschitz condition). Thus, if searching for a picture-book of solutions, best to look elsewhere ! What then do we find in this marvelous resource ?(1) An historical appendix concludes this two-part text: Part one concentrates on the real domain; Part two is the complex domain. Already, then, one realizes that a prerequisite to address the second half will be a smattering of complex variables.(2) Already (page six) partial differentials there to elaborate geometrical aspects (a recurring theme) of a linear differential equation. Jacobians are utilized to fine effect (background prerequisite being some form of advanced calculus). Note the interesting interlude describing Euler's theorem on homogeneous equations (page 10).(3) The usual elementary methods of solution are here in chapter two (pages 16-61) with numerous examples to supplement the prose.(4) I enjoy the "approach from a geometrical point of view" (examples: pages 35, 48 and 55). Excellent !(5) Theory next--that is, third chapter. Notice, again, geometrical viewpoint ever present. Enlightening it all is !(6) Fourth--what a treat at this level--Lie's Theory of continuous transformations (see the example, page 98).Examples abound. A fine mixture of the abstract and concrete. An exciting, eminently accessible, chapter !(7) Theory continues, then onward to chapter six: Linear Equation with Constant Coefficients. This is described in one word: Wronskians. The chapter culminates with brief discussion of behavior at infinity. Exercises are routine.(8) Read next: "it is almost invariably the case that the solution has to be expressed in an infinite form, that is to say, an infinite series, an infinite continued fraction, or a definite integral." (page 159). So begins seventh chapter, which leads one beyond the simplest types of equations solvable by quadrature ( that is: "explicitly integrated by elementary functions"). Simply put, this chapter is essential study for all students. Meet: Legendre functions and asymptotics, too. You are introduced to Bessel functions. Highlight: a connection between differential equations and continued fractions.(9) Laplace transform makes appearance, next (albeit, presented later than many textbooks). This brief chapter (pages 186-203) has fewer examples and will need to be supplemented with a collateral resource. This is followed by another theoretical chapter (Algebraic Theory). Sturm-Liouville systems introduced as prelude to:(10) Boundary Value Problems and Green's functions provides useful examples which ease the burden of theory (page 271). Concluding part one: comparison of Fourier cosine series against Sturm-Liouville (page 276). Part One (Real Domain) finishes after 275 pages, the second half (Complex Domain) is more challenging: I highlight chapter fourteen which deals with non-linear equations, happily, replete with numerous examples. I highlight, too, the interesting discussion "analogies with Fuchsian theory." (page 385). Another gem: Laplace transformations and contour integration (chapter eighteen). The text culminates with a fascinating excursion into the realm of Green's transforms: investigating "the complex zeros of hypergeometric, Bessel, and Legendre functions." Alongside the historical appendix is another detailing numerical methods--elementary and detailed. In conclusion, you will not see 'pretty pictures' and you will hardly be spoon-fed, but, you will arrive at a sound, multifaceted, understanding of ordinary differential equations. Highly recommended for collateral reading.

Readable, has a simple chapter on continuous groups that (implicitly) introduces the notion of global integrability. Discusses and uses Fuch's theorem (classification of singularities of linear ode's, basis for 'guessing' the right form of the series solution in terms of singilarities of coefficients), easy group theoretic discussion of singularities in the complex plane. Stage 2: see Arnol'd's Ordinary Differential Equations for theory, Bender and Orszag for approximation methods.

This classic (originally published in 1926 and still in print!) combines readability with a vast wealth accurately presented material (much of which can still only be found in research papers and certainly can nowhere else be found in a single reference). Most astpects of theory are illustrated by examples.The main areas covered in the book are existence theorems, transformation group (Lie group) methods of solution, linear systems of equations, boundary eigenvalue problems, nature and methods of solution of regular, singular and nonlinear equation in the complex plane, Green's functions for complex equations.This is an essential reference for anyone working with ordinary differential equations.