Project Hub� -Kirchhoff's Law of Voltage (KVL) Mesh Analysis || Science Physics / Electricity Educational Kit(Base-Cardboard)

Descripción

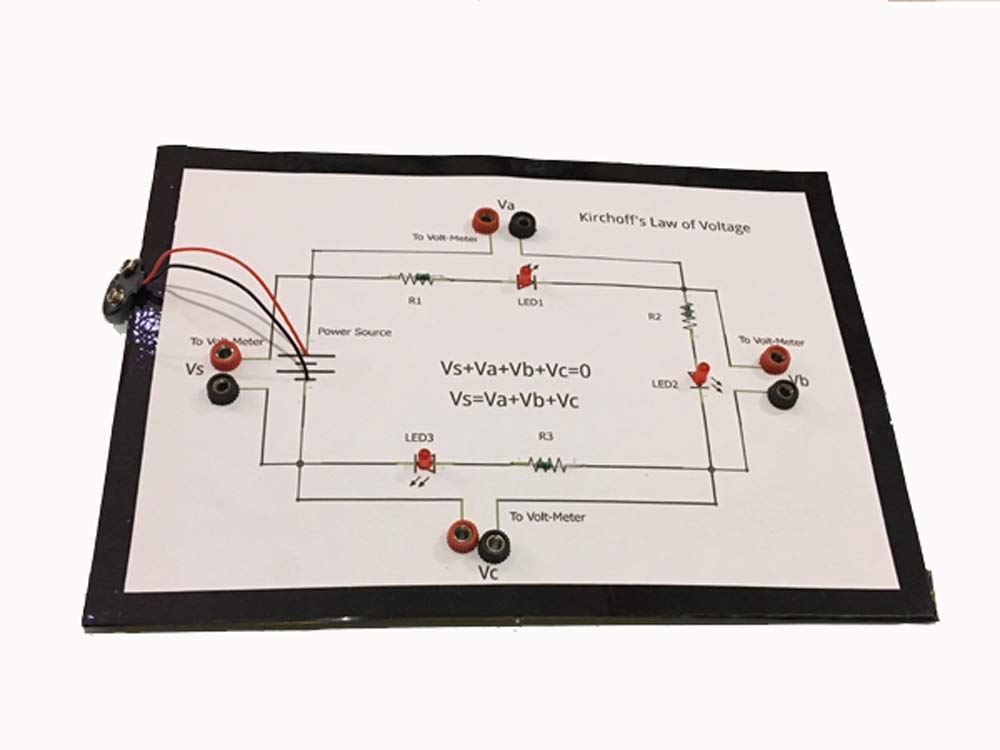
Kirchhoff's Voltage Law (KVL) is Kirchhoff's second law that deals with the conservation of energy around a closed circuit path. His voltage law states that for a closed loop series path the algebraic sum of all the voltages around any closed loop in a circuit is equal to zero. This idea by Kirchhoff is commonly known as the Conservation of Energy, as moving around a closed loop, or circuit, you will end up back to where you started in the circuit and therefore back to the same initial potential with no loss of voltage around the loop. Hence any voltage drops around the loop must be equal to any voltage sources met along the way. So when applying Kirchhoff’s voltage law to a specific circuit element, it is important that we pay special attention to the algebraic signs, (+ and -) of the voltage drops across elements and the emf’s of sources otherwise our calculations may be wrong. This law is also called Kirchhoff's second law, Kirchhoff's loop (or mesh) rule, and Kirchhoff's second rule. The principle of conservation of energy implies that The directed sum of the electrical potential differences (voltage) around any closed network is zero, or: More simply, the sum of the emfs in any closed loop is equivalent to the sum of the potential drops in that loop, or: The algebraic sum of the products of the resistances of the conductors and the currents in them in a closed loop is equal to the total emf available in that loop.